

Visualize adding \(3 + 2\) on the number line by moving from zero three units to the right then another two units to the right, as illustrated below:
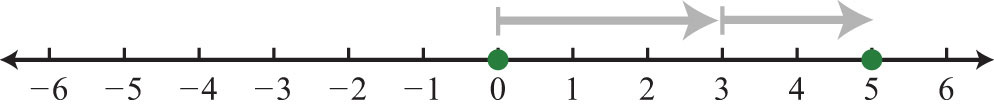
The illustration shows that \(3 + 2 = 5\). Similarly, visualize adding two negative numbers \((−3) + (−2)\) by first moving from the origin three units to the left and then moving another two units to the left.
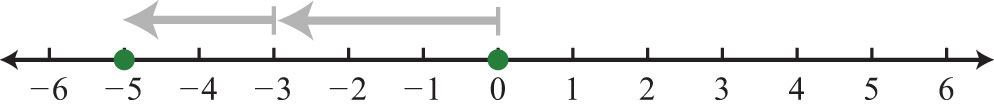
In this example, the illustration shows \((−3) + (−2) = −5\), which leads to the following two properties of real numbers.
Next, we will explore addition of numbers with unlike signs. To add \(3 + (−7)\), first move from the origin three units to the right, then move seven units to the left as shown:
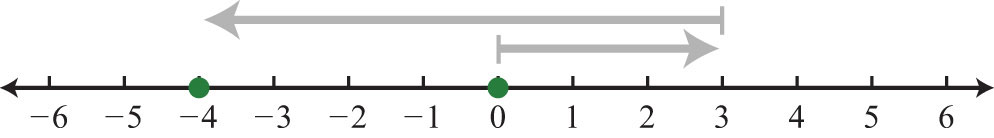
In this case, we can see that adding a negative number is equivalent to subtraction:
It is tempting to say that a positive number plus a negative number is negative, but that is not always true: \(7+(−3)=7−3=4\). The result of adding numbers with unlike signs may be positive or negative. The sign of the result is the same as the sign of the number with the greatest distance from the origin. For example, the following results depend on the sign of the number \(12\) because it is farther from zero than \(5\):
Here \(−25\) is the greater distance from the origin. Therefore, the result is negative.
Answer
Given any real numbers \(a\), \(b\), and \(c\), we have the following properties of addition:
Below are some examples of these properties in action.
a. Adding zero to any real number results in the same real number.
b. Adding opposites results in zero.
Answer
Example \(\PageIndex<3>\)Parentheses group the operations that are to be performed first.
These two examples both result in \(14\): changing the grouping of the numbers does not change the result.
Answer
At this point, we highlight the fact that addition is commutative: the order in which we add does not matter and yields the same result.
On the other hand, subtraction is not commutative.
We will use these properties, along with the double-negative property for real numbers, to perform more involved sequential operations. To simplify things, we will make it a general rule to first replace all sequential operations with either addition or subtraction and then perform each operation in order from left to right.
Replace the sequential operations and then perform them from left to right.
Answer